दोस्तों आज हम अपने ब्लॉगर पर traingles के बारे मे लिखे और अच्छे समझाए भी है आप लोग भी देख सकते है ,दोस्तों अगर आप सभी को मेरे यह ब्लॉगर अच्छा लग रहा है तो प्लीज आप लोग अपने सभी दोस्तों ,सभी ग्रुप पर शेयर ......धन्यवाद ! दोस्तों
Short Trick on Centres of Triangle in Geometry
-------------------------------------------------------------------------------------------------------------
In this article we will discuss about the various centers of triangle. In SSC CGL exams 1 or 2 question generally come in exam from this chapter.
There are four centres in a triangle triangle:
- In-centre
- Circum-centre
- Centroid
- Ortho centre
In-centre:
The point of intersection of the all the three angle bisectors of a triangle is called as In-centre
The distance of the in-centre from the all the three sides is equal(ID=IE=IF=inradius “r”)
∠BIC = 90 +∠A/2In-radius (r)= Area of triangle/Semiperimetre=A/S
∠AIC=90+∠B/2
∠AIB=90+∠C/2
Circumcentre:
The point of intersection of the perpendicular bisectors of the three sides of a triangle is called its circumcentre.
The distance between the circumcentre and the three vertices of a triangle is always equal.
OA=OB =OC=R(circumradius)=abc/4A
∠BOC=2∠A
∠AOC=2∠B
∠AOB=2∠C
Location of circumcentre in various types of triangle:
Acute angle triangle: Lies inside the triangle
Obtuse angle triangle: Lies outside the triangle
Right angle triangle: Lies at the midpoint of the hypotenuse.
Orthocentre:
It is the point of intersection of all the three altitudes of the triangle.
∠BHC=180-∠A
∠AHB=180-∠C
∠AHC=180-∠B
Position of orthocentre inside the triangle:
Acute angled triangle: lies inside the triangle.
Obtuse angle triangle: lies outside the triangle on the backside of the obtuse angle. Orthocentre and circumcentre lie opposite to each other in obtuse angle triangle.
Right angle triangle: lies on the right angle of the triangle.
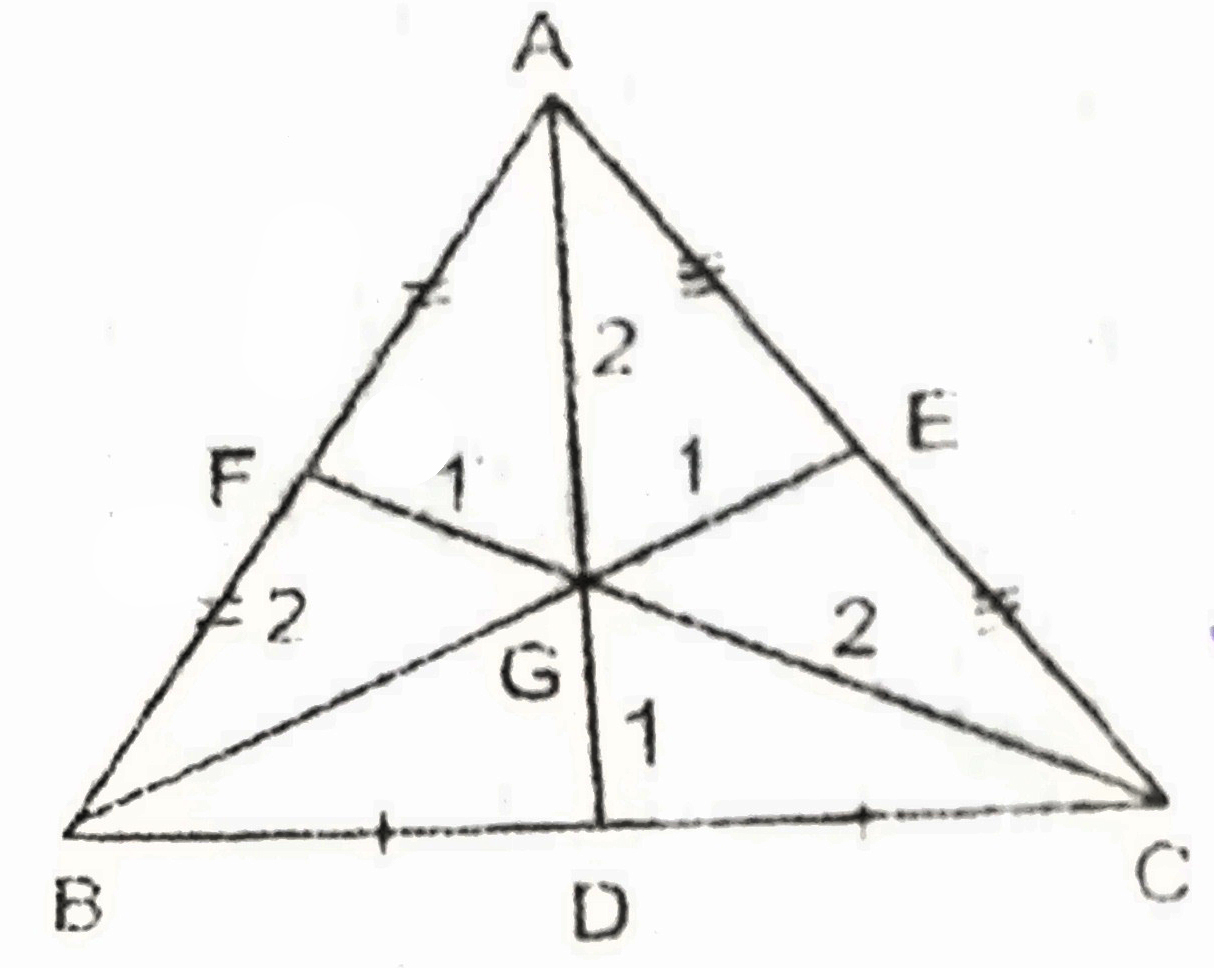
Centriod:
- It is the point of the intersection of the three median of the triangle. It is denoted by G.
- A centroid divides the area of the triangle in exactly three parts.
Medians:
- A line segment joining the midpoint of the side with the opposite vertex is called median.
- Median bisects the opposite side as well as divide the area of the triangle in two equal parts.
Some important tricks are as follows:
(1)In a right angle triangle ABC,∠B=90° & AC is the hypotenuse of the triangle. The perpendicular BD is dropped on the hypotenuse AC from the right angle vertex B,
BD=AB*BC/AC
AD=AB2/AC
CD=BC2/AC
1/BD=1/AB2 +1/BC2
(2)The ratio of the areas of the triangles with equal bases is equal to the ratio of their heights.
(3)The ratio of the areas of two triangles is equal to the ratio of products of base and its corresponding sides.
Area (Triangle ABC)/Area (Triangle PQR) =AC*BD/PR*QS
(4)If two triangles have the same base and lie between the same parallel lines then the area of the two triangles will be equal.
Area of Triangle ABC= Area of Triangle ADB
(5)In a triangle ABC, AE, CD and BF are the medians then
3(AB2+BC2+AC2) = 4(CD2+BF2+AE2)
6)Sum of any two sides of the triangle is always greater than the third sides.
(7)The difference of any two sides of a triangle is always less than the third sides.
Have a nice day
For more info from us:







Nice sir
ReplyDelete